 |
Infinite products with coefficients which vanish on certain arithmetic progressions, International Journal of Number Theory 13(5) (2017), 1095-1117. (with Ayse Alaca, Saban Alaca and Zafer Selcuk Aygin) |
 |
A class of subsums of Euler's sum, Analysis 36(4) (2016), 231-243. |
 |
Ternary quadratic forms and eta quotients, Canadian Mathematical Bulletin 58(4) (2015), 858-868. |
 |
Historical remark on Ramanujan's tau function, American Mathematical Monthly 122(1) (2015), 30-35. |
 |
Double Gauss sums, Journal of Combinatorics and Number Theory 6(2) (2014), 127-153. (with Ayse and Saban Alaca) |
 |
Ternary quadratic forms ax2+by2+cz2 representing all positive integers 8k+4, Acta Arithmetica 166(4) (2014), 391-396. |
 |
On the number of representations of a positive integer as a sum of two binary quadratic forms, International Journal of Number Theory 10(6) (2014), 1395-1420. (with Saban Alaca and Lerna Pehlivan) |
 |
Analogues of Ramanujan's 24 squares formula, International Journal of Number Theory 10(5) (2014), 1099-1119. (with Faruk Uygul) |
 |
The power series expansion of certain infinite products, Ramanujan Journal 33 (2014), 23-53. (with Lerna Pehlivan) |
 |
Euler products in Ramanujan's lost notebook, International Journal of Number Theory 9(5) (2013), 1313-1349. (with Bruce C. Berndt and Byungchan Kim) |
 |
The parents of Jacobi's four squares theorem are unique, American Mathematical Monthly 120(4) (2013), 329-345. |
 |
Quadratic forms and a product-to-sum formula, Acta Arithmetica 158(1) (2013), 79-97. |
 |
On the quaternary forms x2+y2+2z2+3t2, x2+2y2+2z2+6t2, x2+3y2+3z2+6t2 and 2x2+3y2+6z2+6t2, International Journal of Number Theory 8(7) (2012), 1661-1686. (with A. Alaca) |
 |
Some product-to-sum identities, Journal of Combinatorics and Number Theory 4(1) (2012), 35-52. (with S. Alaca and L. Pehlivan) |
 |
Some arithmetic identities involving divisor functions, Functiones et Approximatio 46(2) (2012), 261-271. (with S. Alaca and F. Uygul) |
 |
Fourier series of a class of eta quotients, International Journal of Number Theory 8(4) (2012), 993-1004. |
 |
Restricted Eisenstein series and certain convolution sums, Journal of Combinatorics and Number Theory 3(1) (2011), 1-14. (with A. Alaca, S. Alaca and F. Uygul) |
 |
The number of representations of a positive integer by certain octonary quadratic forms, Functiones et Approximatio 43 (2010), 45-54. (with S. Alaca) |
 |
Sextenary quadratic forms and an identity of Klein and Fricke, International Journal of Number Theory 6 (2010), 169-183. (with A. Alaca and S. Alaca) |
 |
Fourteen octonary quadratic forms, International Journal of Number Theory 6 (2010), 37-50. (with A. Alaca and S. Alaca) |
 |
Representations by sextenary quadratic forms whose coefficients are 1, 2 and 4, Acta Arithmetica 141 (2010), 289-309. (with A. Alaca, S. Alaca and F. Uygul) |
 |
Sums of 4k squares: a polynomial approach, Journal of Combinatorics and Number Theory 1(2) (2009), 33-52. (with A. Alaca and S. Alaca) |
 |
Some infinite products of Ramanujan type, Canadian Mathematical Bulletin 52 (2009), 481-492. (with A. Alaca and S. Alaca) |
 |
Evaluation of the sums Σm=a (mod 4)σ(m)σ(n-m), Czechoslovak Mathematical Journal 59 (2009), 847-859. (with A. Alaca and S. Alaca) |
 |
Some identities involving theta functions, Journal of Number Theory 129 (2009), 1404-1431. (with A. Alaca and S. Alaca) |
 |
Some new theta function identities with applications to sextenary quadratic forms, Journal of Combinatorics and Number Theory 1 (2009), 89-98. (with A. Alaca and S. Alaca) |
 |
The number of representations of a positive integer by certain quaternary quadratic forms, International Journal of Number Theory 5 (2009), 13-40. (with A. Alaca, S. Alaca and M. F. Lemire) |
 |
Seven octonary quadratic forms, Acta Arithmetica 135 (2008), 339-350. (with A. Alaca and S. Alaca) |
 |
Liouville's sextenary quadratic forms x2+y2+z2+t2+2u2+2v2, x2+y2+2z2+2t2+2u2+2v2 and x2+2y2+2z2+2t2+2u2+4v2, Far East Journal of Mathematical Sciences 30 (2008), 547-556.(with A. Alaca and S. Alaca) |
 |
On Liouville's twelve squares theorem, Far East Journal of Mathematical Sciences 29 (2008), 239-242. |
 |
Theta function identities and representations by certain quaternary quadratic forms II, International Mathematical Forum 3 (2008), 539-579. (with A. Alaca, S. Alaca and M. F. Lemire) |
 |
The convolution sum Σl+16m=nσ(l)σ(m), Canadian Mathematical Bulletin 51 (2008), 3-14. (with A. Alaca and S. Alaca) |
 |
Theta function identities and representations by certain quaternary quadratic forms, Int. J. Number Theory 4 (2008), 219-239. (with A. Alaca, S. Alaca and M. F. Lemire) |
 |
Nineteen quaternary quadratic forms, Acta Arithmetica 130 (2007), 277-310. (with A. Alaca, S. Alaca and M. F. Lemire) |
 |
The simplest proof of Jacobi's six squares theorem, Far East J. Math. Sci. 27 (2007), 187-192. (with A. Alaca and S. Alaca) |
 |
On the quaternary forms x2+y2+z2+5t2, x2+y2+5z2+5t2 and x2+5y2+5z2+5t2, JP J. Algebra Number Theory Appl. 9 (2007), 37-53. (with A. Alaca and S. Alaca) |
 |
Jacobi's identity and representation of integers by certain quaternary quadratic forms, Int. J. Modern Math. 2 (2007), 143-176. (with A. Alaca, S. Alaca and M. F. Lemire) |
 |
Evaluation of the convolution sums Σl+24m=nσ(l)σ(m) and Σ3l+8m=nσ(l)σ(m), Math. J. Okayama Univ. 49 (2007), 93-111. (with A. Alaca and S. Alaca) |
 |
On integers with prime factors restricted to certain congruence classes, Far East J. Math. Sci. 24 (2007), 153-161. (with B. K. Spearman) |
 |
Lambert series and Liouville's identities, Dissertationes Math. 445 (2007), 1-72. (with A. Alaca, S. Alaca and E. McAfee) |
 |
An infinite class of identities, Bull. Austral. Math. Soc. 75 (2007), 239-246. (with A. Alaca and S. Alaca) |
 |
Evaluation of the convolution sums Σl+6m=nσ(l)σ(m) and Σ2l+3m=nσ(l)σ(m), J. Number Theory 124 (2007), 491-510. (with S. Alaca) |
 |
Evaluation of the convolution sums Σl+18m=nσ(l)σ(m) and Σ2l+9m=nσ(l)σ(m), International Mathematical Forum 2 (2007), 45-68. (with A. Alaca and S. Alaca) |
 |
The convolution sum Σl+8m=nσ(l)σ(m), Pacific J. Math. 228 (2006), 387-396. |
 |
On a double series of Chan and Ong, Georgian Math. J. 13 (2006), 793-805. |
 |
Evaluation of complete elliptic integrals of the first kind at singular moduli, Taiwanese J. Math. 10, 1633-1660. (with H. Muzaffar) |
 |
The proportion of cyclic quartic field discriminants divisible by a given prime, Kobe J. Math. 23 (2006), 1-10. (with B. K. Spearman) |
 |
Values of the Euler phi function not divisible by a given prime, Kobe J. Math. 23 (2006), 1-10. (with B. K. Spearman) |
 |
On the two-dimensional theta functions of the Borweins, Acta Arith. 124 (2006), 177-195. (with A. Alaca and S. Alaca) |
 |
On the distribution of cyclic cubic fields with index 2, Int. J. Number Theory 2 (2006), 235-247. (with B. K. Spearman) |
 |
Evaluation of the convolution sums ∑ l+12m=n σ(l)σ(m) and ∑ 3l+4m=n σ(l)σ(m), Advances in Theoretical and Applied Mathemeatics 1 (2006), 27-48. (with A. Alaca and S. Alaca) |
 |
Ramanujan identities and Euler products for a type of Dirichlet series, Acta Arith. 122 (2006), 349-393. (with Z.-H. Sun) |
 |
Evaluation of two convolution sums involving the sum of divisors function, Bull. Austral. Math. Soc. 73 (2006), 107-115. (with M. Lemire) |
 |
Evaluation of some convolution sums involving the sum of divisors functions, Yokohama Math. J. 52 (2005), 39-57. (with N. Cheng) |
 |
The convolution sum  , Int. J. Number Theory 1 (2005), 193-205. , Int. J. Number Theory 1 (2005), 193-205. |
 |
A cubic transformation formula for 2F1(1/3,2/3;1;z) and some arithmetic convolution formulae, Math. Proc. Camb. Phil. Soc. 137 (2004), 519-539. |
 |
Density of integers which are discriminants of cyclic fields of odd prime degree, Arch. Math. (Basel) 83 (2004), 507-513. (with B. K. Spearman) |
 |
Evaluation of Weber's functions at quadratic irrationalities, JP J. Algebra Number Theory Appl. 4 (2004), 209-259. (with H. Muzaffar) |
 |
Convolution sums involving the divisor function, Proc. Edinburgh Math. Soc. 47 (2004), 561-572. (with N. Cheng) |
 |
The proportion of cyclic quartic fields with discriminant divisible by a given prime, Proc. Japan Acad. Ser. A Math. Sci. 80 (2004), 141-145. (with B. K. Spearman) |
 |
Density of integers which are discriminants of cyclic cubic fields, Far East J. Math. Sci. 8 (2003), 83-87. (with B. K. Spearman) |
 |
A restricted Epstein zeta function and the evaluation of some definite integrals, Acta Arith. 104 (2002), 23-66. (with H. Muzaffar) |
 |
Integers which are discriminants of bicyclic or cyclic quartic fields, JP J. Algebra Number Theory Appl. 1 (2001), 179-194. (with B.K. Spearman) |
 |
Small solutions of ϕ1x1²+&sdot&sdot&sdot+ϕnxn² =0, Canad. J. Math. 52 (2000), 613-632. (with Z. M. Ou) |
 |
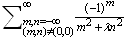 and related series, Mathematical Analysis and Applications (Editor Th. M. Rassias), Hadronic Press, Inc., Florida, 1999, pp. 183-196. (with Zhang Nan-Yue) and related series, Mathematical Analysis and Applications (Editor Th. M. Rassias), Hadronic Press, Inc., Florida, 1999, pp. 183-196. (with Zhang Nan-Yue) |
 |
Some Lambert series expansions of products of theta functions, Ramanujan J. 3 (1999), 367-384. |
 |
Gauss' congruence from Dirichlet's class number formula and generalizations, Number Theory in Progress, Proceedings of the International Conference on Number Theory (Editors: K. Györy, H. Iwaniec and J. Urbanowicz), Zakopane, Poland, 1997, pp. 813-839, deGruyter, Berlin, 1999. (with G. J. Fox and J. Urbanowicz) |
 |
Values of the Dedekind eta function at quadratic irrationalities, Canad. J. Math. 51 (1999), 176-224. (with A. J. van der Poorten) |
 |
On the Epstein zeta function, Tamkang J. Math. 26 (1995), 165-176. (with Zhang Nan-Yue) |
 |
The Chowla-Selberg formula for genera, Acta Arith. 73 (1995), 271-301. (with J. G. Huard and P. Kaplan) |
 |
Comparison of the lengths of the continued fractions of √D and ½(1 + √D), Proc. Amer. Math. Soc. 120 (1994), 995-1002. (with N. Buck) |
 |
Some infinite series involving the Riemann zeta function, in Analysis, Geometry and Groups: A Riemann Legacy Volume, Editors H. M. Srivastava and Th. M. Rassias, Hadronic Press, Palm Harbor, Florida, USA, 1993, pp. 691-712. (with Zhang Nan-Yue) |
 |
Some series representations of zeta(2n+1), Rocky Mountain J. Math. 23 (1993), 1581-1592. (with Zhang Nan-Yue) |
 |
An upper bound for the sum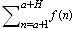 for a certain class of functions ƒ, Proc. Amer. Math. Soc. 114 (1992), 29-35. (with E. Dobrowolski) for a certain class of functions ƒ, Proc. Amer. Math. Soc. 114 (1992), 29-35. (with E. Dobrowolski) |
 |
Evaluation of the infinite series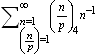 , Proc. Amer. Math. Soc. 109 (1990), 597-603. (with K. Hardy) , Proc. Amer. Math. Soc. 109 (1990), 597-603. (with K. Hardy) |
 |
Determination of all imaginary cyclic quartic fields with class number 2, Trans. Amer. Math. Soc. 311 (1989), 1-55. (with K. Hardy, R. H. Hudson, and D. Richman) |
 |
Congruences modulo 16 for the class numbers of complex quadratic fields, J. Number Theory 27 (1987), 178-195. (with K. Hardy) |
 |
Remark on the class number of Q(√2p) modulo 8 for p≡5(mod 8) a prime, Proc. Amer. Math. Soc. 93 (1985), 198-200. (with C. Friesen) |
 |
Class number formulae of Dirichlet type, Math. Comp. 39 (1982), 725-732. (with R. H. Hudson) |
 |
Congruences modulo 16 for the class numbers of the quadratic fields Q(ñp) and Q(ñ2p) for p a prime congruent to 5 modulo 8, Acta Arith. 40 (1982), 375-397. (with P. Kaplan) |
 |
Congruences modulo 8 for the class numbers of Q(√±p), p ≡ 3 (mod 4) a prime, J. Number Theory 15 (1982), 182-198. |
 |
On the class numbers of Q(√±2p) modulo 16, for p ≡ 1 (mod 8) a prime, Acta Arith. 40 (1982), 289-296. (with P. Kaplan) |
 |
The class number of Q(√p) modulo 4, for p ≡ 5 (mod 8) a prime, Pacific J. Math. 92 (1981), 241-248. |
 |
The class number of Q(√-2p) modulo 8, for p ≡ 5 (mod 8) a prime, Rocky Mountain J. Math. 11 (1981), 19-26. |
 |
On the class number of Q(√-p) modulo 16, for p ≡ 1 (mod 8) a prime, Acta Arith. 39 (1981), 381-398. |
 |
The class number of Q(√-p) modulo 4, for p ≡ 3 (mod 4) a prime, Pacific J. Math. 83 (1979), 565-570. |
 |
Maximal residue difference sets modulo p, Proc. Amer. Math. Soc. 69 (1978), 205-209. (with D. A. Buell) |
 |
Note on integers representable by binary quadratic forms, Canad. Math. Bull. 18 (1975), 123-125. |
 |
Mertens' theorem for arithmetic progressions, J. Number Theory 6 (1974), 353-359. |
 |
The Kloosterman sum revisited, Canad. Math. Bull. 16 (1973), 363-365. |
 |
Exponential sums over GF(2n), Pacific J. Math. 40 (1972), 511-519. |
 |
Small solutions of the congruence a1x1l1 + a2x2l2 + a0 ≡ 0 (mod p), Proc. Cambridge Philos. Soc. 70 (1971), 409-412. |
 |
Note on Salié's sum, Proc. Amer. Math. Soc. 30 (1971), 393-394. |
 |
Note on the number of solutions of f(x1) = f(x2) = ... = f(xr) over a finite field, Canad. Math. Bull. 14 (1971), 429-432. |
 |
Note on the Kloosterman sum, Proc. Amer. Math. Soc. 30 (1971), 61-62. |
 |
On Salié's sum, J. Number Theory 3 (1971), 316-317. |
 |
A distribution property of the solutions of a congruence modulo a large prime, J. Number Theory 3 (1971), 19-32. |
 |
A class of character sums, J. London Math. Soc. 3 (1971), 67-72. |
 |
Asymptotic behaviour of the nth term of certain subsequences of the natural numbers, Delta (Waukesha) 2 (1970), 1-11. |
 |
Finite transformation formulae involving the Legendre symbol, Pacific J. Math. 34 (1970), 559-568. |
 |
Small solutions of the congruence ax2 + by2 ≡ c (mod k), Canad. Math. Bull. 12 (1969), 311-320. |
 |
Note on factorable polynomials, Canad. Math. Bull. 12 (1969), 589-595. |
 |
The distribution of solutions of congruences: Corrigendum and Addendum, Mathematika 16 (1969), 98-100. (with J. H. H. Chalk) |
 |
Distinct values of a polynomial in subsets of a finite field, Canad. J. Math. 21 (1969), 1483-1488. |
 |
Polynomials with irreducible factors of specified degree, Canad. Math. Bull. 12 (1969), 221-223. |
 |
On two conjectures of Chowla, Canad. Math. Bull. 12 (1969), 545-565. |
 |
Note on pairs of consecutive residues of polynomials, Canad. Math. Bull. 11 (1968), 79-83. |
 |
On exceptional polynomials, Canad. Math. Bull. 11 (1968), 279-282. |
 |
Cubic polynomials with the same residues (mod p), Proc. Cambridge Philos. Soc. 64 (1968), 655-658 (with K. McCann). |
 |
The distribution of the residues of a quartic polynomial, Glasgow Math. J. 8 (1967), 67-88. (with K. McCann) |
 |
On extremal polynomials, Canad. Math. Bull. 10 (1967), 585-594. |
 |
On general polynomials, Canad. Math. Bull. 10 (1967), 579-583. |
 |
Pairs of consecutive residues of polynomials, Canad. J. Math. 19 (1967), 655-666. |
 |
The distribution of solutions of congruences, Mathematika 12 (1965), 176-192. (with J. H. H. Chalk) |
 , Int. J. Number Theory 1 (2005), 193-205.
, Int. J. Number Theory 1 (2005), 193-205.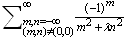 and related series, Mathematical Analysis and Applications (Editor Th. M. Rassias), Hadronic Press, Inc., Florida, 1999, pp. 183-196. (with Zhang Nan-Yue)
and related series, Mathematical Analysis and Applications (Editor Th. M. Rassias), Hadronic Press, Inc., Florida, 1999, pp. 183-196. (with Zhang Nan-Yue)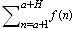 for a certain class of functions ƒ, Proc. Amer. Math. Soc. 114 (1992), 29-35. (with E. Dobrowolski)
for a certain class of functions ƒ, Proc. Amer. Math. Soc. 114 (1992), 29-35. (with E. Dobrowolski)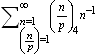 , Proc. Amer. Math. Soc. 109 (1990), 597-603. (with K. Hardy)
, Proc. Amer. Math. Soc. 109 (1990), 597-603. (with K. Hardy)