Polar form (a.k.a trigonometric form)

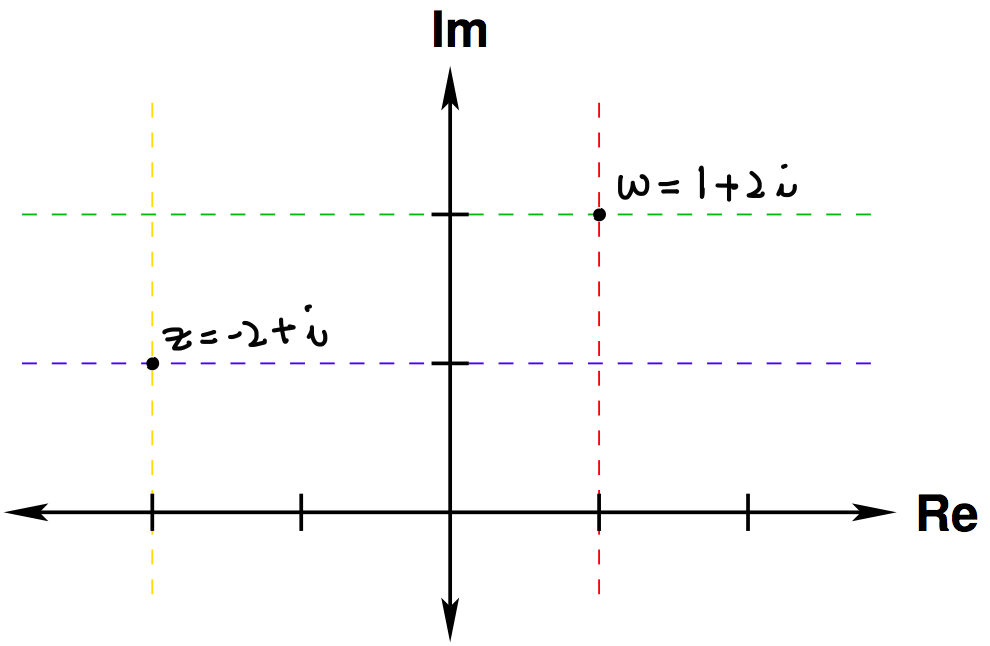
Consider the complex number \(z\) as shown on the complex plane below.

Consider the picture below. The angle that we are interested in is \(\theta\).

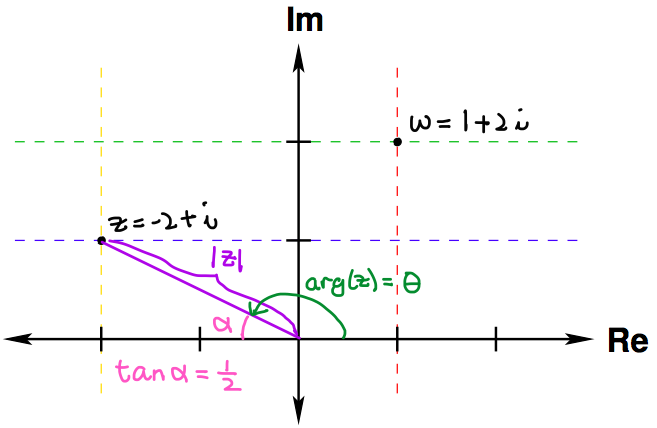
The value of \(\theta\) is \(\pi - \alpha\). Looking at the triangle with vertices at \(z\), \(-2\) and \(0\), we see that \(\tan \alpha = \frac{1}{2}\). So \(\theta = \pi - \arctan \frac{1}{2} = 2.6779\ldots\) radians. (Note that \(\arctan\) is often written as \(\tan^{-1}\).)

A complex number \(z\) in polar form is given as \(r(\cos \theta + i\sin \theta)\) and is often abbreviated as \(r\operatorname{cis} \theta \), where \(r\) equals the modulus of the complex number. The value \(\theta\) is called the argument of \(z\), denoted by \(\operatorname{arg}(z)\).

Note that \(r(\cos (\theta + 2k\pi) + i\sin (\theta + 2k\pi))\) represents the same complex number for every integer \(k\). To achieve uniqueness, it is customary to restrict the argument to be nonnegative and less than \(2\pi\).

For the \(z\) given above, we can write \(z = \sqrt{5} \operatorname{cis} 2.6779\) if we round the argument of \(z\) to 4 decimal places.

To find the polar form of \(w\), first note that \(|~w~| = \sqrt{1^2+2^2} = \sqrt{5}\) and the angle from the positive real axis to the line segment between \(0\) and \(w\) is \(\arctan{2} = 1.1071487...\). Hence, \(w = \sqrt{5}\operatorname{cis} 1.1071\) if we round the argument of \(w\) to 4 decimal places.

In practice, conversion between rectangular form and polar form can only be done approximately (though the accuracy of approximation can be arbitrary) unless the arguments are values of angles whose exact values for sine and cosine are known; e.g. \(\frac{\pi}{6}\), \(\frac{\pi}{4}\), \(\frac{\pi}{3}\), etc.

Multiplying complex numbers in polar form

Let \(z = r_1 \operatorname{cis} \theta_1\) and \(w = r_2 \operatorname{cis} \theta_2\) be complex numbers in polar form. Then \[zw = r_1 r_2 \operatorname{cis} (\theta_1 + \theta_2),\] and if \(r_2 \neq 0\), \[\frac{z}{w} = \frac{r_1}{r_2} \operatorname{cis} (\theta_1 - \theta_2).\]

The first result can prove using the sum formula for cosine and sine. To prove the second result, rewrite \(\displaystyle\frac{z}{w}\) as \(\displaystyle\frac{z\overline{w}}{|~w~|^2}\). The details are left as an exercise.

Examples

1. Let \(z = 4\operatorname{cis} \frac{\pi}{2}\) and \(w = 2 \operatorname{cis} \frac{4\pi}{3}\). Then \begin{eqnarray} \frac{z}{w} & = & \frac{4}{2} \operatorname{cis} \left(\frac{\pi}{2}-\frac{4\pi}{3}\right) \\ & = & 2 \operatorname{cis} \left(-\frac{5\pi}{6}\right) \\ & = & 2 \operatorname{cis} \left(-\frac{5\pi}{6} + 2\pi\right) \\ & = & 2 \operatorname{cis} \frac{7\pi}{6} \end{eqnarray}

2. Let \(z = \operatorname{cis} \theta\) for some \(\theta\). Notice that \(z^2 = \operatorname{cis} (\theta + \theta) = \operatorname{cis} (2\theta)\). Hence, the argument is doubled but the modulus stays the same. And \(z^3 = zz^2 = \operatorname{cis} (\theta + 2\theta) = \operatorname{cis} (3\theta).\) What do you think \(z^{1107}\) is?

Quick Quiz

Exercises

1. Convert each of the following to polar form. Give the exact value for the argument whenever possible; otherwise, give the real and imaginary parts rounded to four decimal places.

   1. \(3\)  

      \(3 \operatorname{cis} 0\)

   2. \(3+4i\)  

      \(5 \operatorname{cis} \left(\arctan\left( \frac{4}{3}\right)\right) \approx 5 \operatorname{cis} 0.9273\)

   3. \(3-4i\)  

      \(5 \operatorname{cis} \left(2\pi - \arctan\left( \frac{4}{3}\right)\right) \approx 5\operatorname{cis} 5.3559\)

   4. \(\displaystyle-2+\sqrt{3}i\)  

      \(\sqrt{7} \operatorname{cis} \left(\pi - \arctan\left( \frac{\sqrt{3}}{2}\right)\right) \approx 2.6458 \operatorname{cis} 2.4279\)

   5. \(2i\)  

      \(2 \operatorname{cis} \frac{\pi}{2}\)

2. Let \(z = r_1 \operatorname{cis} \theta_1\) and \(w = r_2 \operatorname{cis} \theta_2\) be complex numbers in polar form. Show that \[zw = r_1 r_2 \operatorname{cis} (\theta_1 + \theta_2),\] and if \(r_2 \neq 0\), that \[\frac{z}{w} = \frac{r_1}{r_2} \operatorname{cis} (\theta_1 - \theta_2).\]